HAYES RESEARCH GROUP
Saturns Rings
Saturns Rings

Saturn's Rings

Saturns Rings

Despite decades of ground and spacecraft-based observations [2,3,4,5,6], Saturn’s rings still challenge current theories for their origin. In part, this is because their (small) fraction of non-icy material is so poorly characterized. While water ice clearly comprises the bulk of the ring material, the small non-icy fraction is arguably more valuable in revealing the system’s origin [7]. At first, astronomers predominantly agreed Saturn’s rings were primordial [9], until observations from Voyager spacecraft suggested that dynamical consideration necessitated a short (~108yrs) lifetime [1,9,10]. A more recent post-Cassini view returns to an ancient origin [11], but invokes a much more massive pure-ice primordial ring formed via tidal disruption of a Titan-sized differentiated satellite. The abundance of non-icy material can help distinguish between these origin scenarios by constraining the composition of source material and placing an upper limit on the rings’ exposure age to meteoritic bombardment.

At microwave frequencies, the absorptivity of water ice drops dramatically and thermal radiation from the ring plane is dominated by non-icy material. Even if the non-icy material is embedded deeply within thick regions of water ice, thermal photons still have a high probability of outward transmission. In addition, the particle size distribution provides information on dynamical evolution of the ring system. Spacecraft-based telescopes, like Cassini, provide a wide range of geometries, whereas occultation observations are restricted to near-forward scattered radiation. This varied geometry covers scattering angles (angle between the vector from target point to Cassini and the vector from Saturn to target point) from 4° to 160° and constrains the shape of the phase function, which addresses the degree of particle non-sphericity, and can also test occultation measurements of particle size distribution. Furthermore, the sensitivity of radio occultation observations to the size distribution is typically limited to meter scale or large particles [12,15]. Observation of scattered thermal radiation from Saturn, on the other hand, are sensitive to cm-scale particles. The Cassini RADAR radiometer scanned Saturn and its rings on twelve occasions during its prime mission between Dec.2004 – Oct.2008. But despite the obvious advantages of this dataset, the antenna gain function of RADAR, which significantly complicates calibration and processing, make it hard to analyze the observation data.

Step 1: Data Calibration and Sidelobe Removal

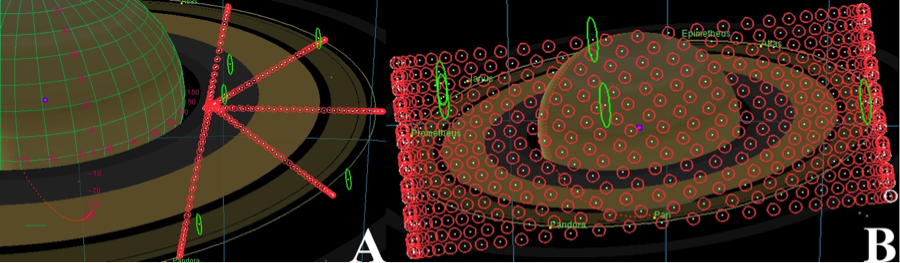
There are two categories of Cassini radiometric observations of Saturn’s rings: low-resolution scans from 20 Saturn radii (Fig.1B) and high-resolution scan from 5-8 Saturn radii (Fig.1A). The nine low-resolution scans were obtained at full linear polarization, ring opening angles from -6° to 22°, and contain both ansae for asymmetry studies. The three high-resolution scans were obtained along specific azimuths at full linear polarization and ring opening angles from 4° to 20°. All told, there are twelve observations available for processing and analysis.

Fig.1 Example of Cassini RADAR observations of Saturns rings

Fig.1 Example of Cassini RADAR observations of Saturn’s rings. High (A) and low (B) resolution scans obtained during Revolution 28 on Sep. 11th and 12th, 2006, respectively. Red circles represent the size of the radiometer’s main beam while the orientations of the green ellipses depict polarization. Two additional scans with 45° offsets in polarization were also obtained

However, this dataset is challenging to process—Saturn and its rings are extended targets with a large dynamic range. Most importantly, each antenna temperature (Ta) measurement represents the convolution of the microwave brightness temperature (Tb) of the target with the radiometer’s gain pattern (G). Moreover Saturn, which is over ten times brighter than the rings, always sits in the sidelobes. Processing the data requires two primary tasks: (1) relate the normalized counts obtained by the radiometer to antenna temperature, and (2) remove beam sidelobe contributions to convert measured antenna temperature to on-axis target brightness temperature. We modified the standard processing algorithms for step (2), originally developed for Titan and Saturn[13,14], to account for the order of magnitude brightness contrast between Saturn and its rings (Tb of 150 K vs.15K). Examples of the preliminary output used to validate this processing are presented in Figs.2 and 3,where we have applied it to a pair of high and low-resolution scans of Saturn’s rings.

Fig.2: Calibrated antenna temp.

Fig.2: Calibrated antenna temp. (A) and best fit brightness temp. model for a low-resolution scan obtained in Sep., 2006 at an inclination (β) of 22°. Data are gridded in the plane perpendicular to the spacecraft-Saturn direction. Spatial position is given in Saturn radii. Note the bright inner B-ring, bright middle C-ring, and forward scattering in the A and B rings as they pass in front of Saturn.

Fig.3: Calibrated antenna temp.

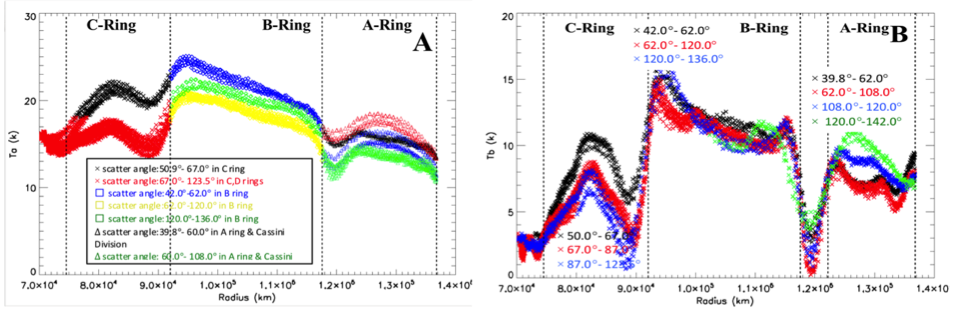
Fig.3: Calibrated antenna temp. (A) and initially processed brightness temp (B) particles for high-res spokes scans acquired in Sep., 2006 at β=20°. Data are plotted against radial distance in the ring plane. Colors depict different scattering angle ranges. Dashed lines show ring boundaries. Note the significant increase in brightness in the central C-Ring and inner B-Ring.

Step 2: Using Thermal Emission to Measure Fraction of Non-Icy Material

In step 1, we got brightness temperature from antenna temperature by removing sidelobe contributions. The derived brightness temperature is composed of scattering component and intrinsic thermal emission. The scatter component is scattered Saturn emission by the ring particles. We currently use a Monte Carlo code [5] to simulate this scattering process. In the code we track the path of each photon before it escapes the rings or gets absorbed by a ring particle. Through the path, the photon can interact with couple ring particles and get either scattered or absorbed, which depends on the particle contamination rate, particle size and the scattering phase function. On the other hand, the intrinsic thermal emission primarily infers the non-icy material fraction mixed in the ring particles. The more contaminated the icy ring particle is, the more absorbing it is and the more intrinsic thermal emission it emits. We derived Saturn’s rings non-icy material fraction by matching observed brightness temperature and Monte Carlo simulation results. The non-icy material fraction is further used to constrain the source composition and provide an integrated history of meteoritic bombardment.

Reference:    

  1. Goldreich and Tremaine (1982), Annual Reviews
  2. Grossman, A.W. 1990, Ph.D. Thesis, Caltech
  3. de Pater and Dickel, 1991, Icarus, 94, 474
  4. van der Tak et al., 1999, Icarus, 142, 125
  5. Dunn, D.E., et al., 2002, Icarus, 160, 132
  6. Dunn, D.E., et al., 2005, Astron. J, 129, 1109
  7. Cuzzi and Estrada (1998), Icarus 132,1
  8. Hedman, M.M. et al. 2011 Science 332,p708
  9. Harris, A.W., et al., 1984, IN: Planetary rings 10.Dones, L., 1991, Icarus 92, 194
  10. Dones, L., 1991, Icarus 92, 194
  11. Canup, R. M., 2010, Nature 468,7326,943
  12. Marouf E.A.et al. 2008“Saturn after Cassini” conf
  13. Janssen, M.A., et al., 2013, Icarus, in-press
  14. Janssen, M.A., et al., 2009, Icarus 200, 222
  15. Marouf, E.A., et al., 1983, Icarus 54, 189